Bouncing Barney

Final Assignment

Faith Hoyt

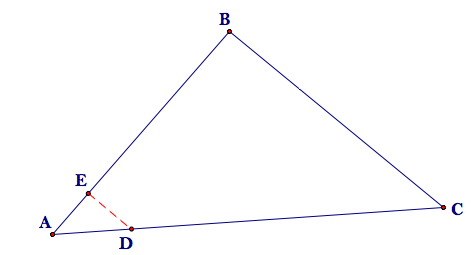
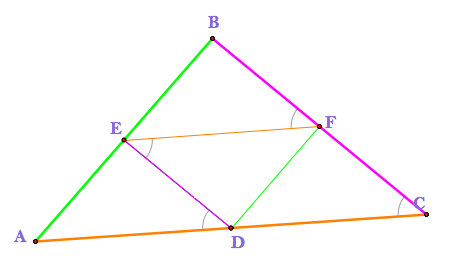
Barney is in a triangular room. He walks from a point on AC parallel to BC. When he reaches AB, he turns and walks parallel to AC. When he reaches BC, he turns and walks parallel to AB. Prove that Barney will eventually return to his starting point. How many times will Barney reach a wall before returning to his starting point?

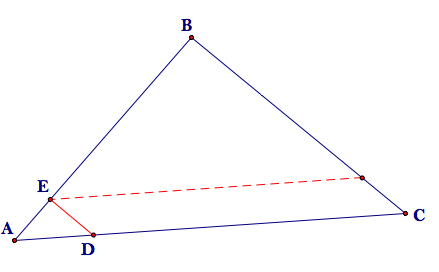
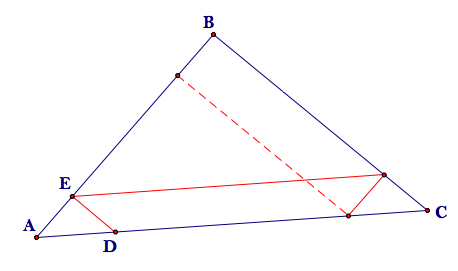
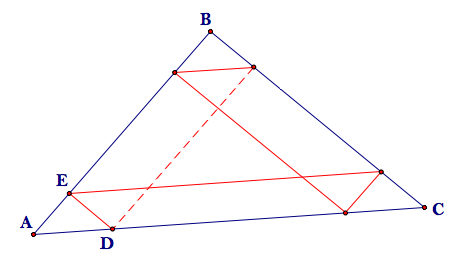
Below is a diagram that shows what we are doing step by step, where the dotted line is his path:

Just by looking at the above pictures, we can see that Barney will reach the wall 5 times before he returns to his starting point. We can also see that when his adventure is complete, he will end up at the same point as where he started.

Now, we need to prove this!

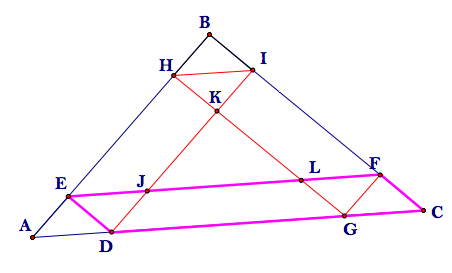
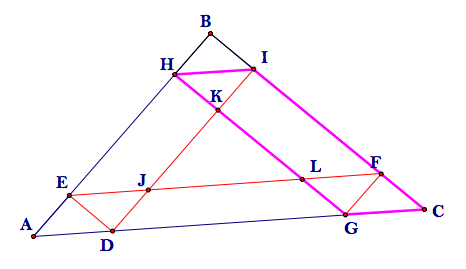
Now, let's look at how far he travels during his expedition. We can verify that this will always be the case by moving our point D.

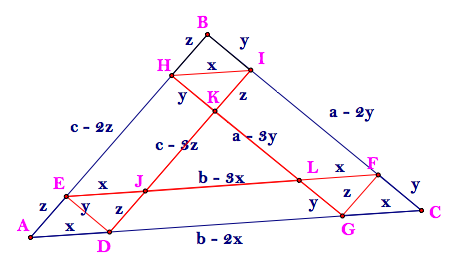
What happens if Barney starts at the midpoint of our bottom segment?

What if Barney starts at one of the trisected points of our bottom segment?

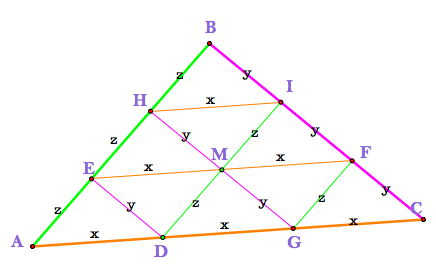
What if Barney starts inside the triangle?

We'll let Barney start at a random point R inside the triangle. Since we are still using parallel lines, we can use the same proof as our first proof. We can also do the same proof for the distance to find that Barney once again travels the same distance as the perimeter of the triangle.

The main difference when Barney starts inside the triangle is that he will hit the wall 6 times before he completes his trip.

What if Barney starts at the orthocenter?

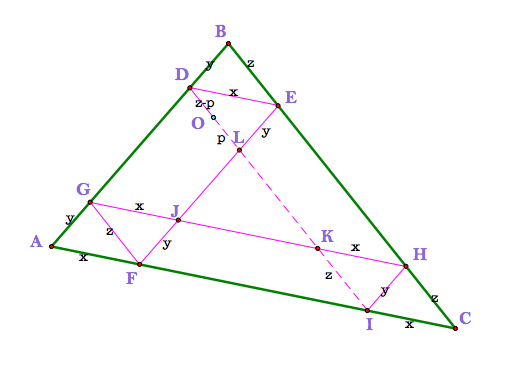
What if Barney starts at some point outside the triangle?

First, we will have to extend our sides. We will also have to make our parallel lines parallel to different sides than we used before.

If we start our point D on the extension of AC, then it will intersect with side BC extended. Our next path will intersect with side AB extended. Our next path will intersect with side AC extended. Our next path will intersect with side BC extended. The next path will intersect with side AB extended. Finally, our last path will intersect back at D on our extension of AC.

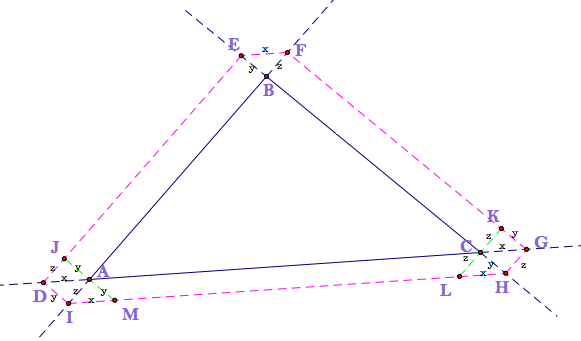
What if Barney starts at a random point outside the triangle that does not lie on the extension of a side?

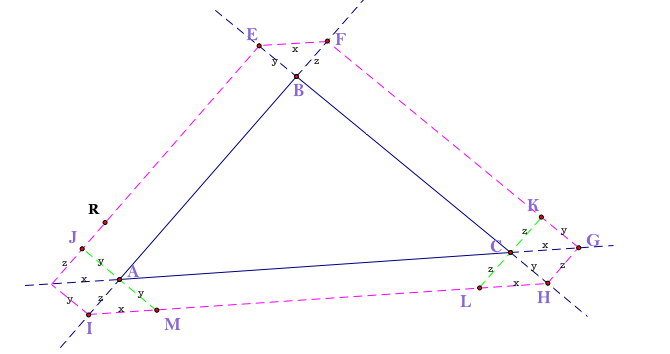
We will place our point R randomly outside the triangle such that it does not lie on the extension of any of our sides.

The difference between our random starting point and starting on the extension of a side is the number of times Barney will hit the wall. In this case, he is going to hit the wall 6 times.

SUMMARY

• Barney will always return to where he started
• If he starts at a point on our starting side, he will return after he has hit the wall 5 times
• If he starts at the midpoint of our starting side, he will return after he has hit the wall twice
• If he starts from a random point inside the triangle, he will return after he has hit the wall 6 times
• If he starts from the centroid or the orthocenter, he will return after he has hit the wall 6 times
• If he starts from a random point outside the triangle, he will return after he has hit the wall 6 times
• The total distance he walks if he starts at the midpoint will be half the perimeter of triangle ABC
• The total distance he walks if he starts outside the triangle will be the sum of the perimeter of triangle ABC plus twice the sum of x, y, and z
• All other starting points will have the total distance he walks equal to that of the perimeter

Possible Extensions?

What if the room is a different shape? A pentagon? Hexagon? Octagon? How will this change our answers about our distance traveled? Will he still end up at the same point he starts at?

Return to my Homepage

Note: Special thanks to my sister, Grace Hoyt, who helped me figure out this proof. :)